Department Urban Water Management
HexCADDIES Hexagonal Grids for urban flood modelling

Hexagonal grids yield two essential advantages over square grids that justify its use for earth surface phenomena, such as overland flow and flood. First, the higher spatial resolution provided by hexagonal grids of the same cell area [Mersereau, 1979]; or, conversely, the fewer number of cells required by an hexagonal grid to match the resolution of a squared counterpart. Second, the hexagonal neighbourhood regularity: in a square grid each cell has four neighbours to which it shares an edge and four to which it shares only a vertex; moreover, neighbour cells in the diagonal are farther apart from the centre. In a regular hexagonal grid each cell has (a) six neighbours to which it shares an edge, and (b) all cell centroids are at the same distance.
In the context of flood modelling neighbourhood regularity makes it especially interesting as flow computations (based on Manning’s equation) use the length of cell edges as the width of the channels connecting the cells. We are currently extending the CADDIES framework (Ghimire et al., 2013) to use hexagonal grids in the cellular automata flood model (CAFlood model). To this end end we are using the HexASCIItypo3/ hexagonal grid file format. Early results of this work will be presented at the Second International Symposium on Cellular Automata Modeling for Urban and Spatial Systems (CAMUSS 2016typo3/).
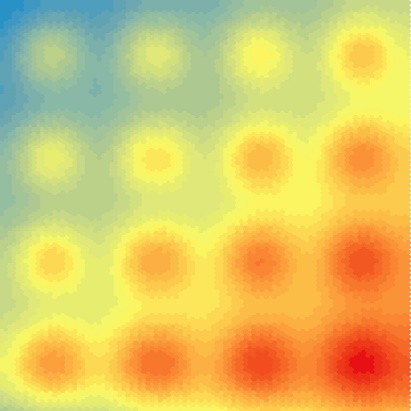
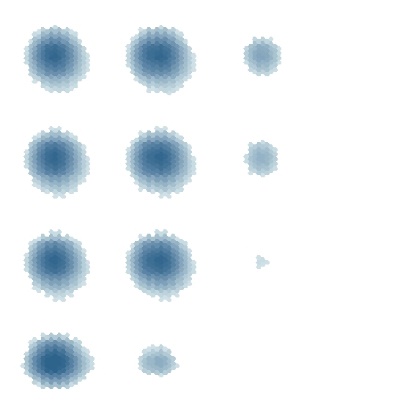
An hexagonal DTM and resulting flood depths produced by CAFlood.
References
de Sousa, L. M., Leitão, J. P. (in preparation). HexASCII: a light text file format for hexagonal grid.
Ghimire, B., Chen, A.S., Guidolin, M., Keedwell, E.C., Djordjević, S., Savić, D.A. (2013). Formulation of a fast 2D urban pluvial flood model using a cellular automata approach. Journal of Hydroinformatics, 15, 676-686. Doi: 10.2166/hydro.2012.245
Mersereau, R.M. (1979). The processing of hexagonally sampled two-dimensional signals. Proceedings of the IEEE, 67(6), 930-949
